-서론-
물론 대수학1을 먼저 정리해야 하지만.. 사실 시간이 너무 부족하고 대수학1은 좀 너무 기초적인 부분이 있다.
그렇다고해서 내가 수학을 마스터 했다는건 아니지만 나중에 복습한다 치고.. 먼저 도움이 당장되는 수학부터 빨리 하고 차근차근 하는게 좋을것 같아 대수학2를 먼저 복습하기로 결정했다.
---------------------------------------------------------------------------------
Intro to Polynomials(다항식 소개)

강사분께서 가볍게 시작하는 마음으로 먼저 Polynomial의 뜻을 풀어주신다. Poly는 Many라는 뜻, 즉 많다고 가볍게 알려주시고 있다.


We are going to talk what are polynomials and what are not polynomials.

노란색 부분들을 Term이라 하며 아마 Term의 여러가지 뜻중 하나인 구간이란 뜻인거 같다.
한국 수학에서는 '항' 이라고 칭한다. ex) 단항식 ,다항식

coefficient 는 계수


You can view this fourth term, or this fourth number, as the coefficient because this could be rewritten as,
instead of just writing as nine, you could write it as nine x to the zero power.( 이런식으로 나타냈을때도 결과는 같으니 4번째 항인 상수도 ,계수라고 칭할수 있다고 설명하고 있다.)


아마도 다항식의 조건이 성립하기 위해서는 상수(coefficients)가 실수가 되어야하고 무리수가 안되어야한다. 그리고
"지수(exponent)" 부분은 음수가 되서는 안되며, 음수가 아닌 정수가 되어야 한다.
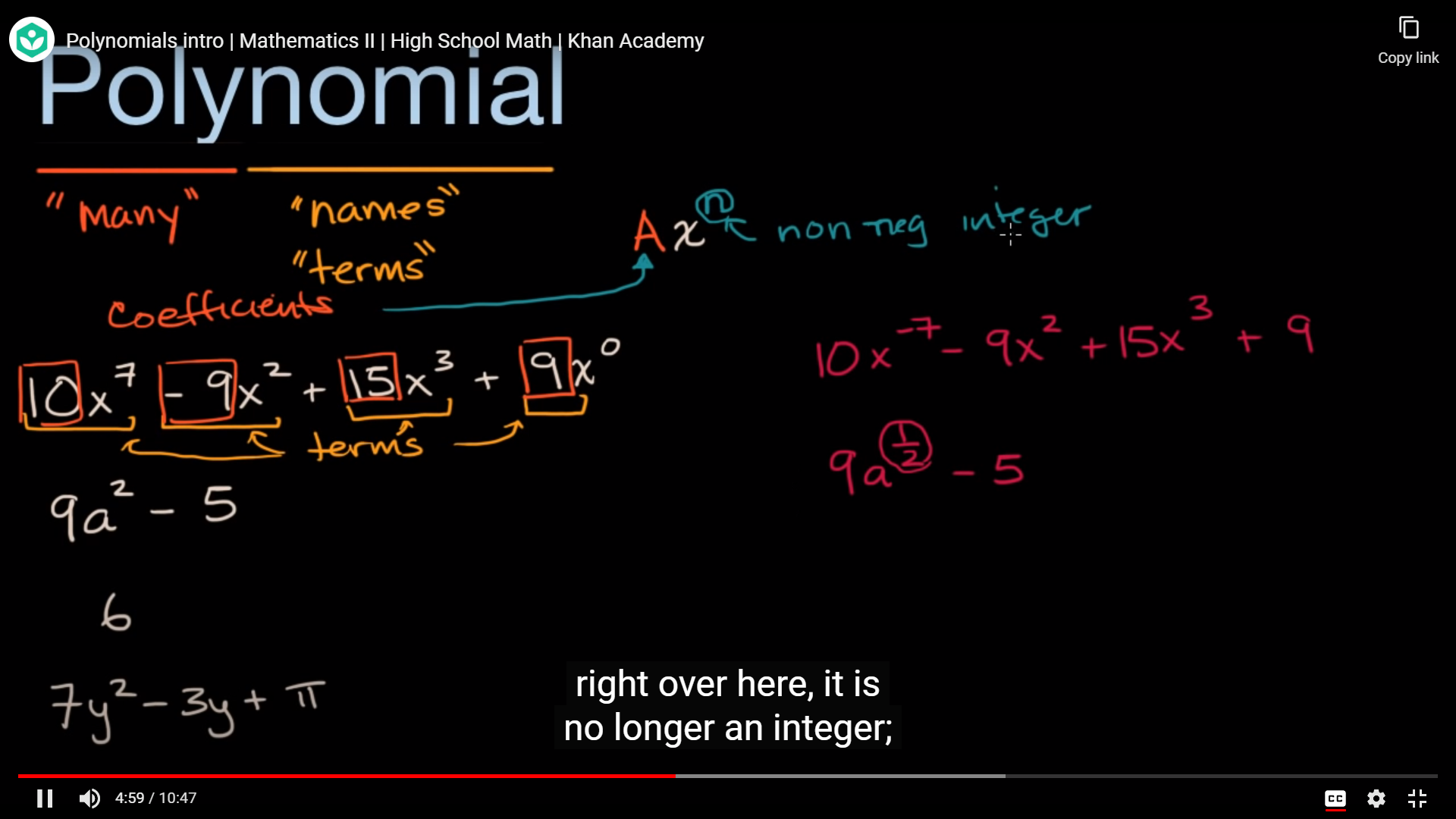
차수(exponent)가 분수가 될수도 없다.


단항식은 영어로 monomial
이항식은 영어로 binomials
binomial has two terms.
Trinomial

Now the next word that you will hear often in the context with polynomials is the notion of the degree of a polynomial.


Degree는 여기서 차수를 의미한다.

what is the degree of the highest term? 여기서는 첫번째 항인 10x to the 7 power 가된다.

The notion of what it means to be leading. It can mean whatever is the first term or the coefficient.
If you are saying leading term, it's the first term. If you are saying leading coefficient,
it is the coefficient in the first term.
(차수가 가장 높은 항을 leading이라하는것 같다.)


Here, it's clear that your leading term is 10x to the seventh, 'cause it's the first one, and our leading coefficient
here is the number 10. So there was a lot in that video, but hopefully the notion of a polynomial isn't seeming
too intimidating at this point.
Standard form 즉 표준상으로 다항식을 표기할때 계수가 높은것 부터 차례대로 표기하라 라는 의미이다.
'Mathematics > Algebra2' 카테고리의 다른 글
| 대 수학2 복소수 2021 05 01 (0) | 2021.05.01 |
|---|---|
| 대수학2 복소수 2021 04 17 (0) | 2021.04.17 |